Welcome to Ask Us Whatever, I’m Joe Sorge.
Today I’m going to explain the outcome of one of the most important and misunderstood experiments involving the speed of light – the Michelson-Morley experiment of 1887. This experiment was designed to reveal the speed at which the Earth travels through what in 1887 was called the luminiferous ether – or something we now can just call “space”.
The experiment did not produce the expected result – in fact, it produced an unexpected null result, suggesting that either the experimental apparatus was not sensitive enough to measure Earth speed, or that there is no medium through which light waves propagate, or that the Earth drags the hypothetical ether with it, or that the Earth does not move through space at all, or that the scientists simply do not fully understand the speed of light.
Which we know has been true up to the present day — until now, of course!
Okay, I described the fundamentals of the Michelson-Morley experiment in Episode 4 so if you’re not familiar with the experiment please review Episode 4 before looking over the rest of this episode. The present episode will essentially be the graduate-level version of Episode 4, and will give us a deeper understanding of the mysteries of light.
In a nutshell, Michelson and Morley sent a light signal along two paths of equal length at right angles from each other. From the perspective of their laboratory, the light appeared to travel the same distance in both directions. But from the perspective of an observer in space, a path that had aligned with the motion of the Earth through space should have covered a longer distance than a path that was at right angles to the direction of the Earth through space.
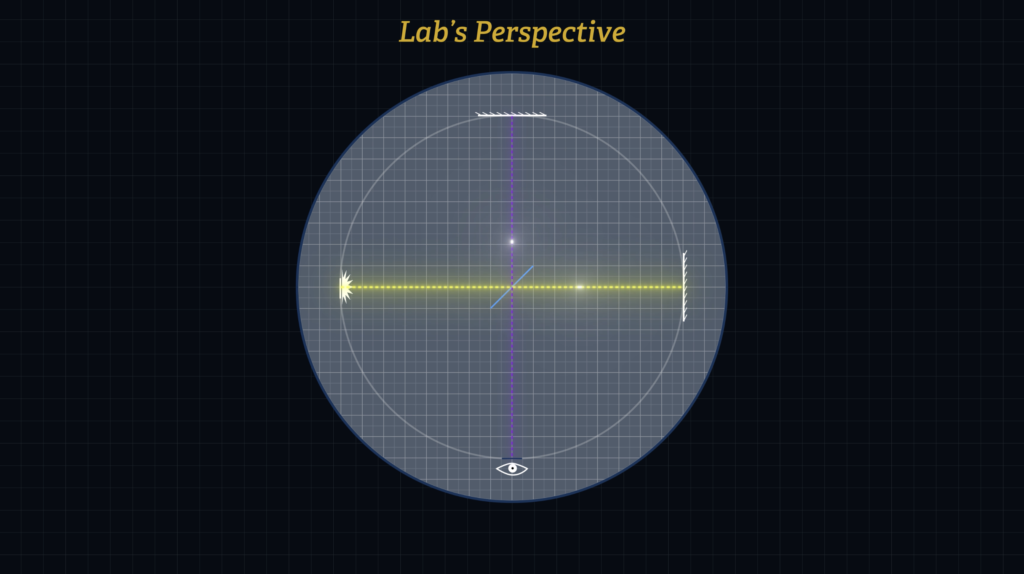

The two light signals were expected to reflect from mirrors and return to the origin at different times, due to the longer path length in the direction of Earth’s motion through space. But they did not observe this – the light signals appeared to return at the same time.
In 1889 George FitzGerald, and later in 1892 Henrik Lorentz suggested that the null result of 1887 could be explained if distances became shorter in the direction of Earth’s motion – something called the Fitzgerald-Lorentz contraction.
They proposed that the longitudinal mirror was drawn closer to the origin of light due to some unknown process, and conveniently by the exact amount that light’s path length would have lengthened in space due to the motion of the Earth.


Now if you’ve watched some other episodes in my video series, you’ll know that I think length contraction is an unworkable idea that creates all types of logical and practical conflicts. But unfortunately, Einstein adopted this concept in his Special Theory of Relativity published in 1905, and later re-emphasized it in 1917. I’d like to emphasize that Einstein did not attribute the length contraction associated with the Michelson-Morley experiment to what is called the relativity of simultaneity, as many textbooks incorrectly suggest.
While Section 2 of his 1905 paper discusses how objects may be perceived to be of different lengths by different observers due to the relativity of simultaneity, Section 4 of the 1905 paper makes it clear that Einstein believed that length contraction was a real, physical phenomenon. He stated in Section 4:
“For v=c all moving objects – viewed from the “stationary” system – shrivel up into plane figures.”
In other words, the x-axis and everything lying along it actually shrink to zero-length. A mere difference in perception due to the relativity of simultaneity cannot account for the Michelson-Morley result. And Einstein’s transformation equations (which are identical to the Lorentz transforms) incorporated the hypothetical physical contraction of distances into the math, not merely a difference in perception.


It’s remarkable that Einstein incorporated length contraction into his Special Theory of Relativity. I mean, Einstein did not like the idea of a luminiferous ether and so he eliminated it from Special Relativity. Yet he clung fervently to the idea of a constant light speed, even though light speed does not need to be constant if you eliminate the notion of an ether from the model for how light propagates through space. Einstein essentially threw out the baby but didn’t throw out the bathwater.
So, let’s tackle the Michelson-Morley result without relying on length contraction, and let’s do this for any angle at which the Earth might be whizzing or twisting through space relative to the two arms of the Michelson-Morley apparatus.
Let’s also include the presence or absence of a refractive medium so that we can better understand some experimental data that I discussed in Episode 9.4.
I am about to show you how light can appear to travel at a constant speed in all directions in a moving reference frame, such as the Earth, which preserves the constancy of light speed implied by Maxwell’s equations, yet at the same time allowing light to travel at direction-dependent speeds through space. In other words, traveling faster than speed c through space while appearing to all observers in all moving reference frames to travel at speed c in all directions within their reference frames. Sound impossible? Watch!
We’ll begin from the perspective of observers on the Earth, which we will deem to be our moving Frame S’.
What do we know about light speed on the Earth? Well, it appears to be constant in all directions. And if we pass light through a refractive medium, such as water or glass, it also appears to travel at a constant but slower speed in all directions, regardless of the time of day or the season of the year. In other words, it seems to be independent of the motion of the Earth through space.
If we quantify light’s speed through refractive media by measuring the ratio of light speed through vacuum on the Earth divided by light speed through the refractive medium on the Earth, we call the ratio of the two, the refractive index for the medium, “n”.
\(\frac{c_{\text {vacuum }}^{\prime}}{c^{\prime}{ }_{\text {medium }}}=n_{\text {refractive index }} \)
I use the term c-prime in the ratio to remind us that these speeds are being measured on the moving Earth. The value for n is about 1.333 for water, and about 1.5 for glass, although both measurements can vary with temperature, wavelength, additives, et cetera.
\begin{gathered} n_{\text {water }}=1.333 \\ n_{\text {glass }}=1.5 \end{gathered}
It will be equal to c’ divided by n times the cosine of the angle formed between the x’-axis and the direction of a light ray as measured from the perspective of Frame S’, an angle I call phi-prime-sub-n.
\(u_x^{\prime}=\frac{c^{\prime}}{n} \cos \phi_n^{\prime} \text { meters } / \text { second }{ }^{\prime} \)

And so, we do not need to label Earth meters with a prime symbol. But since atomic clocks on the Earth will beat at a different tempo than identical atomic clocks that are stationary in space, we keep track of units of time on the Earth using the prime symbol.
\(u_x^{\prime}=\frac{c^{\prime}}{n} \cos \phi_n^{\prime} \text { meters } / \text { second }{ }^{\prime} \)
Now my alternative model, covered in Episode 6.1, provides transforms for both dx’ and dt’ using information gathered from the stationary Frame S of space, where gamma(s) is the square root of one plus v squared over c squared.
\(d x^{\prime}=d x-v d t \)
\(d t^{\prime}=\gamma_s d t-\frac{v d x}{\gamma_S c^2} \)
\(\gamma_s=\sqrt{1+v^2 / c^2} \)
If we equate the transforms for dx’ divided by dt’ to c’ over n times cosine of phi-prime-sub-n,
\(\frac{d x^{\prime}}{d t^{\prime}}=\frac{d x-v d t}{\gamma_s d t-\frac{v d x}{\gamma_s c^2}}=\frac{c^{\prime}}{n} \cos \phi_n^{\prime} \text { meters } / \text { second }{ }^{\prime} \)
and then carry out a series of tedious algebraic steps that I’m not going to display but you can verify, we obtain the formula that we derived in Episode 9.4 for speed dx/dt through stationary space, which we call u-sub-x for short.
\(u_{x(\text { alt })}=\frac{d x}{d t}=\frac{c^{\prime}}{\gamma_s n \pm \frac{v}{c} \cos \phi_n^{\prime}} \cos \phi_n^{\prime} \pm v \text { meters/second } \)
c’ divided by gamma-s times n plus or minus v over c times the cosine of phi-prime-sub-n all times the cosine of phi-prime-sub-n plus or minus v.
In other words, if an observer in Frame S’ shines a light at angle phi-prime-sub-n relative to the direction of the movement of the Earth, then the x-component of light speed observed by someone in Frame S will be described by this formula.
\(u_{x(a l t)}=\frac{d x}{d t}=\frac{c^{\prime}}{\gamma_s n \pm \frac{v}{c} \cos \phi_n^{\prime}} \cos \phi_n^{\prime} \color{#FF0000}{ \pm v} \text { meters/second } \)
As pointed out in Episode 9.4, what is so remarkable about this formula is that the isolated speed v reflects the speed of the source of the light relative to Frame S.
In other words, contrary to what is preached about Special Relativity, light speed coming from a moving source when observed from Frame S is affected by the speed of its emitter. The formula for the x-component of longitudinal light speed through space, when n = 1 and the cosine of phi-prime-sub-n is 1, as observed from Frame S, will produce a speed that is greater than speed c when the source is moving at speed v through Frame S.
\(\phi_n^{\prime}=0^{\circ} \quad n_{\text {vacuum }}=1 \quad \cos \phi_n^{\prime}=1 \)
\(u_{x(\text { alt })}=\frac{c^{\prime}}{\gamma_s n \pm \frac{v}{c} \cos \phi_n^{\prime}} \cos \phi_n^{\prime} \pm v=\frac{c^{\prime}}{\gamma_s \pm \frac{v}{c}} \pm v=\gamma_s c \text { meters/second } \)

Just as an aside, the Special Relativity version of this formula requires only slight modifications, where the Lorentz gamma factor is used instead of gamma-s, and the placement of these gamma factors forces u-sub-x to equal c when n equals 1 and the cosine of phi-prime-sub-n equals 1.
\( \phi_n^{\prime}=0^{\circ} \quad n_{\text {vacuum }}=1 \quad \cos \phi_n^{\prime}=1 \)
\(u_{x(\text { Special Relativity })}=\frac{\frac{c^{\prime}}{\gamma_L}}{\gamma_L n \pm \gamma_L \frac{v}{c} \cos \phi_n^{\prime}} \cos \phi_n^{\prime} \pm v=\frac{\frac{c^{\prime}}{\gamma_L}}{\gamma_L \pm \gamma_L \frac{v}{c}} \pm v=c \text { meters/second } \)
In other words, Einstein used the Fitzgerald-Lorentz length contraction fudge factor, an extra Lorentz gamma factor in the numerator, to force a constant longitudinal light speed of c in Frame S for any value of speed v; even though there was no experimental evidence that light must travel at speed c in Frame S when emitted by a source that is moving at speed v through Frame S, and even though he had abandoned the idea of a speed-defining luminiferous ether.
Regardless, note that this Special Relativity version of my formula also contains an isolated plus or minus v term indicating that the speed of the source of the light signal also plays an important role in the overall speed of the light signal as observed from Frame S. We’ll come back to that.
\(u_{x(\text { Special Relativity })}=\frac{\frac{c^{\prime}}{\gamma_L}}{\gamma_L n \pm \gamma_L \frac{v}{c} \cos \phi_n^{\prime}} \cos \phi_n^{\prime} \color{#FF0000}{ \pm v}=\frac{\frac{c^{\prime}}{\gamma_L}}{\gamma_L \pm \gamma_L \frac{v}{c}} \color{#FF0000}{ \pm v}=c \text { meters/second } \)
OK, how do these formulas impact the Michelson-Morley result?
Well, if we just run the alternative model transformations in reverse, the x-component of light speed in Frame S, u-sub-x, is transformed into its speed in the moving frame, u-prime-sub-x, which produces, a constant light speed of c’ divided by the refractive index, n, times the cosine of phi-prime-sub-n as measured on the Earth, in perfect agreement with empirical data.
\(u_x^{\prime}=\frac{d x^{\prime}}{d t^{\prime}}=\frac{d x \mp v d t}{\gamma_s d t \mp \frac{v d x}{\gamma_s c^2}}=\frac{u_x \mp v}{\gamma_s \mp \frac{v u_x}{\gamma_s c^2}}=\frac{c^{\prime}}{n} \cos \phi_n^{\prime} \text { meters } / \text { second }^{\prime} \)
That is, even if light speed as observed from Frame S is equal to gamma(s) times c in the vacuum of space, it will be observed as speed c in vacuum on the Earth. I cannot overemphasize this.
And it does not matter whether light is emitted parallel to the direction of the motion of the emitting source,
\(u_{x(a l t \color{#4fa72d}{+v})}=\frac{c^{\prime}}{\gamma_s+\frac{v}{c}}+v=\gamma_s c \text { meters } / \text { second } \)
or anti-parallel to the direction of motion of the emitting source.
\(u_{x(a l t \color{#FF0000}{-v})}=\frac{c^{\prime}}{\gamma_s-\frac{v}{c}}-v=\gamma_s c \text { meters } / \text { second } \)
Light speed in both longitudinal directions will be gamma-s times c through Frame S. The speed of the emitter, v, when added or subtracted to or from the intrinsic speed of light as measured with Frame S clocks, will produce an overall light speed that exceeds speed c. But when the speed of this same light signal is measured by observers in Frame S’, using clocks of Frame S’ that have been synchronized with a Frame S’ light signal, lightspeed will be measured to travel through vacuum in Frame S’ at speed c.
19th and 20th century physicists were geocentric creatures. They measured light speed on the Earth using Earth-based measuring tools, and they eventually came up with a speed of 299,792,458 meters per Earth second’. There is no fundamental reason why the speed of light traveling through one reference frame must be numerically identical when measured from a different reference frame using the measuring tools of that different reference frame.
The same is true for other phenomena involving the measurement of time. If the clocks of different reference frames can beat at different tempos, then time-dependent parameters, such as speed, acceleration, force, et cetera can also produce different numerical values when measured with such different clocks.
OK, let’s look at the y’-component of speed. This is equal to, c’ divided by n times the sine of phi-prime-sub-n in Frame S’.
\(u_y^{\prime}=\frac{d y^{\prime}}{d t^{\prime}}=\frac{c^{\prime}}{n} \sin \phi_n^{\prime} \text { meters } / \text { second }{ }^{\prime} \)
The transform to solve for dy’/dt’ using Frame S quantities is as follows.
\(\frac{d y^{\prime}}{d t^{\prime}}=u_{y(a l t)}^{\prime}=\frac{d y}{\gamma_s d t-\frac{v d x}{\gamma_s c^2}}=\frac{u_y}{\gamma_s-\frac{v u_x}{\gamma_s c^2}}=\frac{c^{\prime}}{n} \sin \phi_n^{\prime} \text { meters } / \text { second }{ }^{\prime} \)
If we solve for u-sub-y we obtain, c’ over gamma-s times n plus or minus v over c times the cosine of phi-prime-sub-n all times the sine of phi-prime-sub-n.
\(u_{y(a l t)}=\frac{d y}{d t}=\frac{c^{\prime}}{\gamma_s n \pm \frac{v}{c} \cos \phi_n^{\prime}} \sin \phi_n^{\prime} \text { meters } / \text { second } \)
When light is aimed 90 degrees from the direction of Earth’s motion through space, the sine of phi-prime-sub-n is equal to 1, the cosine is equal to 0, n equals 1 in vacuum, and u-sub-y equals c divided by gamma-s.
\(\phi_n^{\prime}=90^{\circ} \quad \sin \phi_n^{\prime}=1 \quad \cos \phi_n^{\prime}=0 \quad n_{\text {vacuum }}=1 \)
\(u_{y(a l t)}=\frac{c^{\prime}}{\gamma_s n \pm \frac{v}{c} \cos \phi_n^{\prime}} \sin \phi_n^{\prime}=\frac{c^{\prime}}{\gamma_s} \text { meters } / \text { second } \)
\(\gamma_S=\sqrt{1+\frac{v^2}{c^2}} \)
As an aside, the equivalent Special Relativity formula yields a value for u-sub-y equal to c divided by the Lorentz gamma factor.
\(u_{y(\text { Special Relativity })}=\frac{c^{\prime}}{\gamma_L n \pm \frac{v}{c} \cos \phi_n^{\prime}} \sin \phi_n^{\prime}=\frac{c^{\prime}}{\gamma_L} \text { meters } / \text { second } \)
\(\gamma_L=\sqrt{\frac{1}{1-\frac{v^2}{c^2}}} \)
The formulas are almost identical since Einstein did not incorporate length contraction along the y-axis.
These formulas have enormous implications for light traveling from distant stars that are moving toward, or away, or at odd angles with respect to the light signal traveling toward the Earth.
According to my model, a light signal coming from a star that is moving either directly toward or directly away from the Earth will travel at speed gamma-s times c. And a light signal coming from a star that is moving transverse to the direction of the light signal will travel at a speed comprised of both transverse and longitudinal velocity components.
Now it’s important to recognize that a Michelson-Morley result requires the constancy of round-trip travel times in both Frames S’ and S.
In other words, while the amount of time required to travel from the beam splitter to either mirror need not equal the amount of time required to return from such mirror back to the beam splitter; the amount of time required to travel the round-trip distance from beam splitter to either mirror and then back again to beam splitter must be the same in both Frames S and S’ for both arms of the Michelson-Morley apparatus, regardless of the direction in which the Earth is traveling through space. In other words, regardless of the value of phi-prime-sub-n.

Since empirical measurements on the Earth have demonstrated that light speed over identical Frame S’ distances are always the same, regardless of time of day or orientation of the light signal with respect to Earth’s orbit, then Frame S’ travel times must be the same in all directions.
\(d t^{\prime}{ }_{\text {all directions }}=\frac{n}{c^{\prime}} d x^{\prime}=\text { constant } \)
The question then is whether this is true or not in Frame S. The distance traveled for a given light signal in Frame S will be, the square root of the sum of the squares of the x- and y-components of distance traveled outbound or distance traveled inbound,
\(d r_{\text {outbound }}=\sqrt{d x_{\text {out }}^2+d y_{\text {out }}^2} \)
\(d r_{\text {inbound }}=\sqrt{d x_{\text {in }}^2+d y_{\text {in }}^2} \)
which we can derive from the transforms.
\(d x_{\text {out }}=\gamma_s^2 d x^{\prime}+\gamma_s v d t^{\prime} \)
\(d x_{\text {in }}=-\gamma_s^2 d x^{\prime}+\gamma_s v d t^{\prime} \)
\(d y_{\text {out}}= d y^{\prime} \)
\(d y_{\text {in}}= -d y^{\prime} \)
Therefore, average travel time is equal to, the mean of the outbound distance traveled divided by the outbound speed plus the inbound distance traveled divided by the inbound speed.
\(d t_{\text {average }}=\frac{1}{2}\left(\frac{d r_{\text {out }}}{u_{\text {out }}}+\frac{d r_{\text {in }}}{u_{\text {in }}}\right)=\frac{d r_{\text {average }}}{u_{\text {time-weighted average }}}=\frac{d r_{\text {average }} *\left(d t_{\text {out }}+d t_{\text {in }}\right)}{\left(u_{\text {out }} d t_{\text {out }}+u_{\text {in }} d t_{\text {in }}\right)} \)
This is also equal to the average distance traveled divided by the time-weighted speed.
If this number is constant for all values of phi-prime-sub-n, then light signals will re-join at the beam splitter at the same time, and thus fail to create a fringe shift. And this, in fact, is what is observed whenever a Michelson-Morley type experiment is performed.

If we were to work through the algebra, we would find that round trip time dt is always constant regardless of the angle between the light signal and Earth’s motion through space. That time is equal to two times gamma-s times dt’.
\(d t_{\text {round trip}} = d t_{\text{out}} + d t_{\text {in}} \)
\(d t_{\text {round trip }}=\gamma_s d t^{\prime}+\frac{v d x^{\prime}}{c^2}+\gamma_s d t^{\prime}-\frac{v d x^{\prime}}{c^2} \)
\(d t_{\text {round trip }}=2 \gamma_s d t^{\prime} \)
\(\gamma_S=\sqrt{1+\frac{v^2}{c^2}} \)
Gamma-s varies only with speed v, which will remain constant during each leg of the round-trip path from beam splitter to mirror and back; and dt’ varies only with the refractive index n, and the length of the Michelson-Morley apparatus arms dx’, which are held constant during the experiment.
\(d t_{\text {all directons }}^{\prime}=\frac{n}{c^{\prime}} d x^{\prime}=\text { constant } \)
Therefore, both light signals will complete the round-trip paths in exactly the same amount of time in Frame S, regardless of the orientation of the arms of the Michelson-Morley apparatus with respect to the motion of the Earth.
OK, let’s try to sum this up with some take-home statements.
When light is emitted through vacuum, 90 degrees to the direction of Earth’s motion, it travels in the y-direction at speed c divided by gamma(s) along a round trip distance of 2 times dy’.
And so, the time required, expressed in Frame S units is, 2 times gamma-s times dt’ seconds.
\(d t_{\text {average }}=\frac{2 d x^{\prime}}{\frac{c}{\gamma_s}}=\frac{2 \gamma_s d x^{\prime}}{c}=2 \gamma_s d t^{\prime} \text { seconds } \)
And when light is emitted parallel to the direction of Earth’s motion, it travels in the x-direction at a faster speed, gamma-s times c, but the average path length is longer than dx’ by a factor of gamma-s-squared. And so, the time required, expressed in Frame S units is, also, two times gamma-s times dt’ seconds.
\(d t_{\text {average }}=\frac{2 \gamma_s^2 d x^{\prime}}{\gamma_s c}=\frac{2 \gamma_s d x^{\prime}}{c}=2 \gamma_s d t^{\prime} \text { seconds } \)
And when the angles with respect to the direction of Earth’s motion are in between 90 degrees and 0 degrees, light speeds and round-trip path lengths change, but the changes remain proportional and so the round-trip travel times remain the same.

You should now understand why Michelson and Morley obtained a null result regardless of the positioning of their apparatus, the time of day, or the season of the year. Experiments performed on the Earth, where the light source and measuring instruments are traveling together with the Earth through space, will always yield a light speed of c’ meters per second’. In other words, 299,792,458 meters per Earth second’.
Although Michelson and Morley failed to achieve their goal of measuring the speed of the Earth through space, there is still hope – but it involves performing some experiments in space rather than on the Earth. Such experiments will be covered in Episode 8.2.
Alright, well, that’s it for now. If you have any questions, please leave them in the comments section. I’m Joe Sorge, and thanks for watching.