Welcome to Ask Us Whatever. I’m your host, Joe Sorge.
You know, most of us have become reliant on the GPS systems in our smartphones to get places.
But did you know that without the adjustments to time and space predicted by Einstein’s relativity theories, our phones would take us to the wrong locations?

That motivated me to try to understand relativity. What a mistake!?
You’ve seen those Hollywood movies about slowing the aging process by traveling quickly through space. It used to seem far-fetched to me, and whenever I asked someone to explain it, I either got a blank stare, or a bunch of equations and a recitation of textbook doctrine. It never made any sense. So, I decided to study it and break it down in a relatable way so that you all can understand it. Can we really slow down aging by traveling rapidly? Well, I think the answer is yes, but unexpectedly not exactly by the amount that Einstein proposed.
The goal of this video series on relativity is, first, to explain the concepts in a way that makes sense; then answer some deeper questions about the meaning and implications; and then finally, to correct some problems with special relativity. That’s right, I have the audacity to think that I’ve found some flaws in Einstein’s equations, and that I know how to fix them. You can be the judge.
Let’s start by describing a version of relativity that Albert Einstein first developed in the year 1905, called special relativity. In a nutshell, special relativity concerns itself with the study of objects that move in straight lines, and at constant speeds. These objects are said to be in “inertial reference frames”, which means you can think of them like objects floating in space.

And when these objects need to communicate, Einstein recognized that the time required for those communications to travel from one location to the other could confuse things. Communication requires a means of transmission, such as sound, or radio waves, or light, or smoke signals (which really operate on light). And since these forms of communication move at certain speeds, there will be delays between the sending and receiving of the signals.
For example, it takes a little under 5 seconds for sound to travel to an observer witnessing a lightning strike one mile away. That’s because sound travels much slower than light. Yet, an observer who is much closer to the lightning strike will see the lightning and hear the thunder with much less delay in between. But since light travels extremely fast, it’s difficult to comprehend the impact of a light-based delay.
So, rather than using light to introduce the concepts behind special relativity, I’m going to start with an unconventional approach: I’m going to use sound and music instead. The properties of sound not only can teach us how certain aspects of special relativity work, but will also give us some deep insight into the “reality” versus “illusions” of special relativity.
Now, most of us have noticed that the pitch of a siren changes as it passes us. It’s called the Doppler effect. And although the pitch of the sound changes as the siren comes and goes, the speed at which the sound travels through air doesn’t, regardless of whether the sound comes from an oncoming siren or a retreating siren.

This concept of speed as a property governed by the mediumthrough which “waves” are traveling, independent of the source of the waves, is one of the fundamental principles of special relativity. Except Einstein didn’t believe that light needed any medium at all to propagate. According to Einstein, light just had its own, constant speed because…it just did. We’ll get to that in a later episode.
But sound needs air to propagate, and it travels through air at approximately 1125 feet per second, or about 770 miles per hour under typical conditions. That’s faster than most jets fly, but not by much.

Let’s play with that for a bit. Imagine you’ve snuck into an outdoor concert venue, the day before the big event. You’re standing right up against the mainstage while a band is practicing. Not wanting to pass up the opportunity to do a thought-experiment, you decide to pull out your smartphone and measure the amount of time between beats.
And you see that a full second passes in between each beat, and since our imaginary band is playing one beat every second, they are playing at a “tempo” of 60 beats per minute.
Suddenly, imaginary security guards pull up in an imaginary ATV, and ask for your credentials, which you don’t have because this thought experiment wasn’t well planned out. So, they whisk you away very quickly, at precisely 77 miles per hour, which just coincidentally happens to be one tenth the speed of sound.

Now as you hang on for your life, you notice that the pitch of the music drops, like the band has decided to play in a different key and their tempo has slowed down.
You look at your smartphone, and you notice that the beats are no longer hitting at increments of each full second, they’re now occurring somewhere in between the full seconds. And, the beats are each lasting longer than a second. In fact, you now hear only 9 beats for every 10 seconds being displayed on your smartphone. You and the band are experiencing musical time differently.
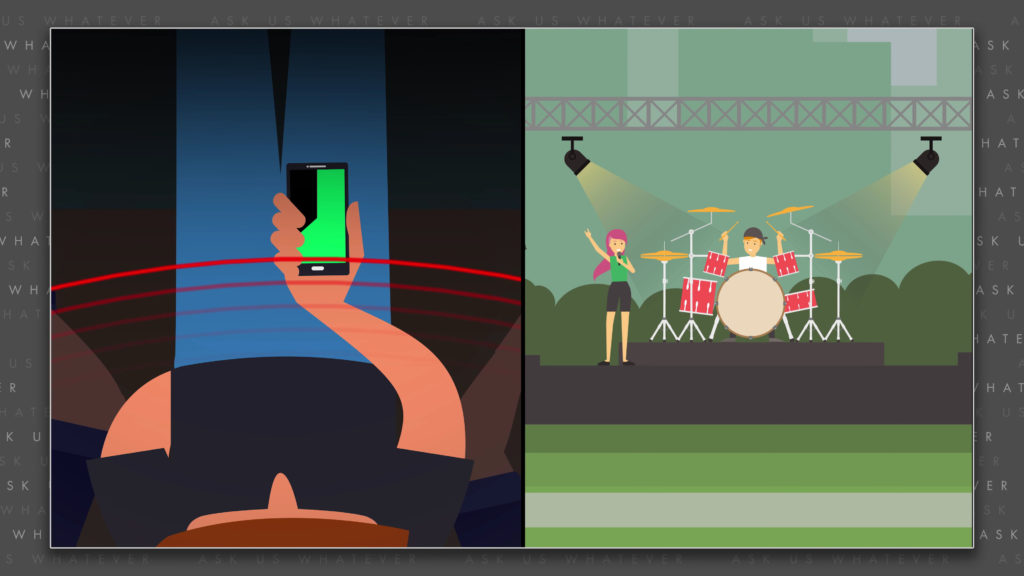
Before your ride with the security guards, you experienced those 9 beats in 9 seconds. But now that you are moving away from the sound waves, causing each wave to travel farther and farther to reach you, it takes 10 seconds for the 9 beats to reach your ears.
What if you didn’t have a smartphone with you, or any type of clock? What if the time between each beat was your sole measure of time; in fact, it was everyone’s measure of time? What if, instead of a musical band, some large loudspeakers belted out the sound of a clocking ticking; and the people in the town used that tick-tock-tick-tock tempo to calibrate their clocks?
Well people standing still might define one minute differently than people traveling away from the town in a train. Their concepts of time would be different. Their definitions of a minute would be different. This is not the result of special relativity, which also alters time, but it’s a great illustration of how a method to measure time, “the tempo of a periodic rhythm”, can change depending on one’s state of motion.
Now what happens when the security guards stop the ATV at the exit gate to evict you, and you’re no longer moving relative to the musicians?
You notice that the pitch of the music rises to the original key, and the tempo returns to one beat every second. You look at your smartphone to confirm that you are once again hearing 1 beat per second, but you notice something strange. The beats have remained out of sync with the flashing of the full second digit on your phone.
Your journey has uncoupled the synchronization between your phone and the band. And the effect was real, and it didn’t reverse itself when the ATV stopped. It’s like you traveled through a time warp, and emerged on the other side in a slightly shifted world.

You know what would happen if you traveled back, from the exit toward the stage? Well, the pitch of the music you would hear would actually go higher than the pitch being played by the band, and the tempo would increase to a rate actually faster than the tempo of the band. And if you traveled at exactly the same speed and over exactly the same path back to the band, you will have reversed the time warp.
Again, this is not due to special relativity. But it does illustrate how our perception of time can change depending on our state of motion.
Okay, in Episode 2 we’ll cover how motion can change our perceptions of simultaneous events.
Thanks for watching.